دانشنامه ریاضی و کامپیوتر
سایت جامع در باب کتب و جزوات رشته های ریاضی و کامپیوتر با دانلود مستقیم.
سایت جامع در باب کتب و جزوات رشته های ریاضی و کامپیوتر با دانلود مستقیم.
 |
 |
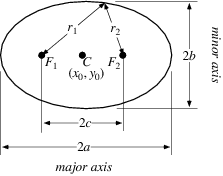
An ellipse is a curve that is the locus of all points in the plane the sum of whose distances ![]() and
and ![]() from two fixed points
from two fixed points ![]() and
and ![]() (the foci) separated by a distance of
(the foci) separated by a distance of ![]() is a given positive constant
is a given positive constant ![]() (Hilbert and Cohn-Vossen 1999, p. 2). This results in the two-center bipolar coordinate equation
(Hilbert and Cohn-Vossen 1999, p. 2). This results in the two-center bipolar coordinate equation
|
(1)
|
where ![]() is the semimajor axis and the origin of the coordinate system is at one of the foci. The corresponding parameter
is the semimajor axis and the origin of the coordinate system is at one of the foci. The corresponding parameter ![]() is known as the semiminor axis.
is known as the semiminor axis.
The ellipse is a conic section and a Lissajous curve.
An ellipse can be specified in Mathematica using Circle[![]() x, y
x, y![]() ,
, ![]() a, b
a, b![]() ].
].
If the endpoints of a segment are moved along two intersecting lines, a fixed point on the segment (or on the line that prolongs it) describes an arc of an ellipse. This is known as the trammel construction of an ellipse (Eves 1965, p. 177).

It is possible to construct elliptical gears that rotate smoothly against one another (Brown 1871, pp. 14-15; Reuleaux and Kennedy 1876, p. 70; Clark and Downward 1930; KMODDL).
The ellipse was first studied by Menaechmus, investigated by Euclid, and named by Apollonius. The focus and conic section directrix of an ellipse were considered by Pappus. In 1602, Kepler believed that the orbit of Mars was oval; he later discovered that it was an ellipse with the Sun at one focus. In fact, Kepler introduced the word "focus" and published his discovery in 1609. In 1705 Halley showed that the comet now named after him moved in an elliptical orbit around the Sun (MacTutor Archive). An ellipse rotated about its minor axis gives an oblate spheroid, while an ellipse rotated about its major axis gives a prolate spheroid.
A ray of light passing through a focus will pass through the other focus after a single bounce (Hilbert and Cohn-Vossen 1999, p. 3). Reflections not passing through a focus will be tangent to a confocal hyperbola or ellipse, depending on whether the ray passes between the foci or not.
Let an ellipse lie along the x-axis and find the equation of the figure (1) where ![]() and
and ![]() are at
are at ![]() and
and ![]() . In Cartesian coordinates,
. In Cartesian coordinates,
|
(2)
|
Bring the second term to the right side and square both sides,
|
(3)
|
Now solve for the square root term and simplify
|
(4)
|
|||
|
(5)
|
|||
|
(6)
|
Square one final time to clear the remaining square root,
|
(7)
|
Grouping the ![]() terms then gives
terms then gives
|
(8)
|
which can be written in the simple form
|
(9)
|
Defining a new constant
|
(10)
|
puts the equation in the particularly simple form
|
(11)
|
The parameter ![]() is called the semiminor axis by analogy with the parameter
is called the semiminor axis by analogy with the parameter ![]() , which is called the semimajor axis (assuming
, which is called the semimajor axis (assuming ![]() ). The fact that
). The fact that ![]() as defined above is actually the semiminor axis is easily shown by letting
as defined above is actually the semiminor axis is easily shown by letting ![]() and
and ![]() be equal. Then two right triangles are produced, each with hypotenuse
be equal. Then two right triangles are produced, each with hypotenuse ![]() , base
, base ![]() , and height
, and height ![]() . Since the largest distance along the minor axis will be achieved at this point,
. Since the largest distance along the minor axis will be achieved at this point, ![]() is indeed the semiminor axis.
is indeed the semiminor axis.
If, instead of being centered at (0, 0), the center of the ellipse is at (![]() ,
, ![]() ), equation (◇) becomes
), equation (◇) becomes
|
(12)
|
As can be seen from the Cartesian equation for the ellipse, the curve can also be given by a simple parametric form analogous to that of a circle, but with the ![]() and
and ![]() coordinates having different scalings,
coordinates having different scalings,
|
(13)
|
|||
|
(14)
|
The general quadratic curve
|
(15)
|
is an ellipse when, after defining
 |
(16)
|
||
|
(17)
|
|||
|
(18)
|
![]() ,
, ![]() , and
, and ![]() . Also assume the ellipse is nondegenerate (i.e., it is not a circle, so
. Also assume the ellipse is nondegenerate (i.e., it is not a circle, so ![]() , and we have already established is not a point, since
, and we have already established is not a point, since ![]() ). In that case, the center of the ellipse
). In that case, the center of the ellipse ![]() is given by
is given by
|
(19)
|
|||
|
(20)
|
the semi-axes lengths are
![sqrt((2(af^2+cd^2+gb^2-2bdf-acg))/((b^2-ac)[sqrt((a-c)^2+4b^2)-(a+c)]))](http://mathworld.wolfram.com/images/equations/Ellipse/Inline70.gif) |
(21)
|
||
![sqrt((2(af^2+cd^2+gb^2-2bdf-acg))/((b^2-ac)[-sqrt((a-c)^2+4b^2)-(a+c)])).](http://mathworld.wolfram.com/images/equations/Ellipse/Inline73.gif) |
(22)
|
and the counterclockwise angle of rotation from the ![]() -axis to the major axis of the ellipse is
-axis to the major axis of the ellipse is
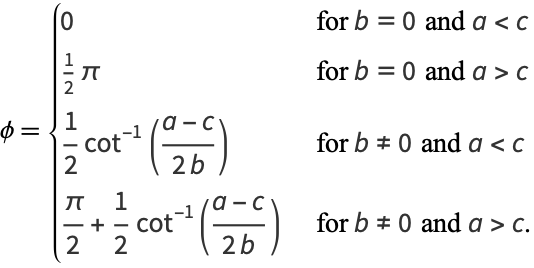 |
(23)
|
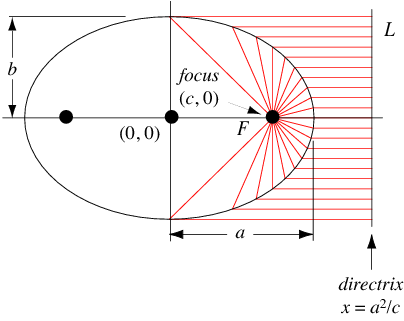
The ellipse can also be defined as the locus of points whose distance from the focus is proportional to the horizontal distance from a vertical line known as the conic section directrix, where the ratio is ![]() . Letting
. Letting ![]() be the ratio and
be the ratio and ![]() the distance from the center at which the directrix lies, then in order for this to be true, it must hold at the extremes of the major and minor axes, so
the distance from the center at which the directrix lies, then in order for this to be true, it must hold at the extremes of the major and minor axes, so
|
(24)
|
Solving gives
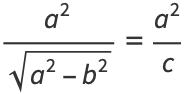 |
(25)
|
||
|
(26)
|
The focal parameter of the ellipse is
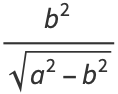 |
(27)
|
||
|
(28)
|
|||
|
(29)
|
where ![]() is a characteristic of the ellipse known as the eccentricity, to be defined shortly.
is a characteristic of the ellipse known as the eccentricity, to be defined shortly.
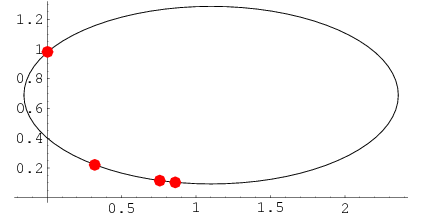
An ellipse whose axes are parallel to the coordinate axes is uniquely determined by any four non-concyclic points on it, and the ellipse passing through the four points ![]() ,
, ![]() ,
, ![]() , and
, and ![]() has equation
has equation
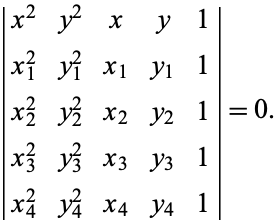 |
(30)
|
Let four points on an ellipse with axes parallel to the coordinate axes have angular coordinates ![]() for
for ![]() , 2, 3, and 4. Such points are concyclic when
, 2, 3, and 4. Such points are concyclic when
|
(31)
|
where the intermediate variable ![]() has been defined (Berger et al. 1984; Trott 2006, pp. 39-40). Rather surprisingly, this same relationship results after simplification of the above where
has been defined (Berger et al. 1984; Trott 2006, pp. 39-40). Rather surprisingly, this same relationship results after simplification of the above where ![]() is now interpreted as
is now interpreted as ![]() . An equivalent, but more complicated, condition is given by
. An equivalent, but more complicated, condition is given by
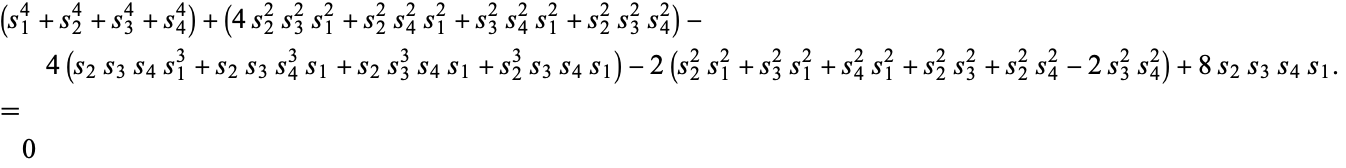 |
(32)
|
Like hyperbolas, noncircular ellipses have two distinct foci and two associated directrices, each conic section directrix being perpendicular to the line joining the two foci (Eves 1965, p. 275).
Define a new constant ![]() called the eccentricity (where
called the eccentricity (where ![]() is the case of a circle) to replace
is the case of a circle) to replace ![]()
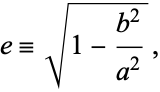 |
(33)
|
from which it follows that
|
(34)
|
|||
|
(35)
|
|||
|
(36)
|
|||
|
(37)
|
|||
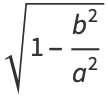 |
(38)
|
||
|
(39)
|
The eccentricity can therefore be interpreted as the position of the focus as a fraction of the semimajor axis.
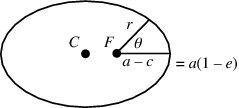
If ![]() and
and ![]() are measured from a focus
are measured from a focus ![]() instead of from the center
instead of from the center ![]() (as they commonly are in orbital mechanics) then the equation s of the ellipse are
(as they commonly are in orbital mechanics) then the equation s of the ellipse are
|
(40)
|
|||
|
(41)
|
and (◇) becomes
|
(42)
|
Clearing the denominators gives
|
(43)
|
Substituting in ![]() gives
gives
|
(44)
|
Plugging in to re-express ![]() and
and ![]() in terms of
in terms of ![]() and
and ![]() ,
,
|
(45)
|
Dividing by ![]() and simplifying gives
and simplifying gives
|
(46)
|
which can be solved for ![]() to obtain
to obtain
|
(47)
|
The sign can be determined by requiring that ![]() must be positive. When
must be positive. When ![]() , (47) becomes
, (47) becomes ![]() , but since
, but since ![]() is always positive, we must take the negative sign, so (47) becomes
is always positive, we must take the negative sign, so (47) becomes
|
(48)
|
|
(49)
|
|
(50)
|
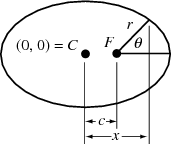
The distance from a focus to a point with horizontal coordinate ![]() (where the origin is taken to lie at the center of the ellipse) is found from
(where the origin is taken to lie at the center of the ellipse) is found from
|
(51)
|
Plugging this into (50) yields
|
(52)
|
|
(53)
|
In pedal coordinates with the pedal point at the focus, the equation of the ellipse is
|
(54)
|
The arc length of the ellipse is
|
(55)
|
|||
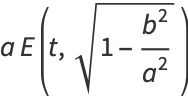 |
(56)
|
||
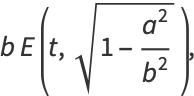 |
(57)
|
where ![]() is an incomplete elliptic integral of the second kind with elliptic modulus
is an incomplete elliptic integral of the second kind with elliptic modulus ![]() (the eccentricity).
(the eccentricity).
The relationship between the polar angle from the ellipse center ![]() and the parameter
and the parameter ![]() follows from
follows from
|
(58)
|
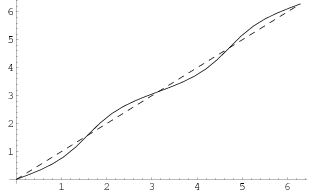
This function is illustrated above with ![]() shown as the solid curve and
shown as the solid curve and ![]() as the dashed, with
as the dashed, with ![]() . Care must be taken to make sure that the correct branch of the inverse tangent function is used. As can be seen,
. Care must be taken to make sure that the correct branch of the inverse tangent function is used. As can be seen, ![]() weaves back and forth around
weaves back and forth around ![]() , with crossings occurring at multiples of
, with crossings occurring at multiples of ![]() . The curvature and tangential angle of the ellipse are given by
. The curvature and tangential angle of the ellipse are given by
|
(59)
|
|||
|
(60)
|
The entire perimeter ![]() of the ellipse is given by setting
of the ellipse is given by setting ![]() (corresponding to
(corresponding to ![]() ), which is equivalent to four times the length of one of the ellipse's quadrants,
), which is equivalent to four times the length of one of the ellipse's quadrants,
|
(61)
|
|||
|
(62)
|
|||
|
(63)
|
where ![]() is a complete elliptic integral of the second kind with elliptic modulus
is a complete elliptic integral of the second kind with elliptic modulus ![]() (the eccentricity). The perimeter can be computed using the rapidly converging Gauss-Kummer series as
(the eccentricity). The perimeter can be computed using the rapidly converging Gauss-Kummer series as
|
(64)
|
|||
|
(65)
|
(Sloane's A056981 and A056982), where ![]() is a binomial coefficient and
is a binomial coefficient and
|
(66)
|
This can also be written analytically as
|
(67)
|
|||
|
(68)
|
where ![]() is a hypergeometric function,
is a hypergeometric function, ![]() is a complete elliptic integral of the first kind.
is a complete elliptic integral of the first kind.