A cubic curve invented by Diocles in about 180 BC in connection with his attempt to duplicate the cube by geometrical methods. The name "cissoid" first appears in the work of Geminus about 100 years later. Fermat and Roberval constructed the tangent in 1634. From a given point there are either one or three tangents to the cissoid.
Given an origin 
and a point 
on the curve, let 
be the point where the extension of the line 
intersects the line 
and 
be the intersection of the circle of radius 
and center 
with the extension of 
. Then the cissoid of Diocles is the curve which satisfies 
.
The cissoid of Diocles is the roulette of a parabola vertex of a parabola rolling on an equal parabola. Newton gave a method of drawing the cissoid of Diocles using two line segments of equal length at right angles. If they are moved so that one line always passes through a fixed point and the end of the other line segment slides along a straight line, then the midpoint of the sliding line segment traces out a cissoid of Diocles.
The cissoid of Diocles is given by the parametric equation s
 |
 |
 |
(1)
|
 |
 |
 |
(2)
|
for 
. Converting these to polar coordinates gives
 |
(3)
|
As an implicit equation ,
 |
(4)
|
which is equivalent to
 |
(5)
|
It has a cusp at the origin, and vertical asymptote at 
.
As found by Huygens and Wallis in 1658, the area between the curve and its vertical asymptote is
 |
 |
 |
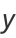(6)
|
 |
 |
 |
(7)
|
(MacTutor Archive).
In this parametrization, the arc length, curvature, and tangential angle are given by
 |
 |
![a[-4+sqrt(3)ln2+2sqrt(3)ln(2+sqrt(3))-2sqrt(3)ln[sqrt(6)cost+sqrt(5+3cos(2t))]+sqrt(10+6cos(2t))sect]](http://mathworld.wolfram.com/images/equations/CissoidofDiocles/Inline27.gif) |
(8)
|
 |
 |
 |
(9)
|
 |
 |
 |
(10)
|
for 
and 
.
An alternative parametric form is
 |
 |
 |
(11)
|
 |
 |
 |
(12)
|
(Gray 1997) for 
. In this parametrization, the arc length, curvature, and tangential angle are
 |
 |
![2a[sqrt(t^2+4)-2+sqrt(3)tanh^(-1)(2/(sqrt(3)))-sqrt(3)tanh^(-1)(sqrt((t^2+4)/3))]](http://mathworld.wolfram.com/images/equations/CissoidofDiocles/Inline45.gif) |
(13)
|
 |
 |
 |
(14)
|
 |
 |
 |
(15)
|
for 
and 
.
SEE ALSO: Cissoid, Cissoid of Diocles Catacaustic, Cissoid of Diocles Inverse Curve, Cissoid of Diocles Pedal Curve, Ophiuride
REFERENCES:
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 214, 1987.
Gray, A. "The Cissoid of Diocles." §3.5 in Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed. Boca Raton, FL: CRC Press, pp. 57-61, 1997.
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 98-100, 1972.
Lockwood, E. H. A Book of Curves. Cambridge, England: Cambridge University Press, pp. 130-133, 1967.
MacTutor History of Mathematics Archive. "Cissoid of Diocles." http://www-groups.dcs.st-and.ac.uk/~history/Curves/Cissoid.html.
Smith, D. E. History of Mathematics, Vol. 2: Special Topics of Elementary Mathematics. New York: Dover, p. 327, 1958.
Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. Middlesex, England: Penguin Books, p. 34, 1986.
Yates, R. C. "Cissoid." A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 26-30, 1952.
